In the currency market, interest rates are a key component that influences traders and investors sentiments, and consequently, fuel price fluctuations. Traders and investors analyze them to gauge how a given fiat should trade or exchange against others in the trillion-dollar marketplace.
Decisions carried by some of the biggest central banks led by the Federal Reserve and the European Central bank around borrowing charges are a key component that influences price movements in the forex market. Investors and market makers pay close attention to bank rates when it comes to placing trades.
By simply looking at the prime rates between two nations, it becomes pretty easy to tabulate a future exchange duty between two legal tenders. Interest rate parity (IRP) is the equation that is commonly used to compute future interchange tariffs.
Understanding IRP
Interest rate parity tries to show or govern the relationship between a country’s annual percentage rate and prevailing currency conversion ratio. The metric affirms a strong connection between fiat values and the bank tariff.
With this theory, it does not matter if a person invests money in one country and then converts the returns to another fiat or convert first and then invest overseas. The fact that there is a connection between the forward exchange toll and lending levy makes it possible to generate the same amount regardless of whether one invests first and then converts or converts first and then invests.
Therefore, the IRP equitation is commonly used to calculate future interchange ratios duties at present.
The metric is especially useful when dealing with currencies of two countries with different interest rates. In this case, it becomes pretty easy to compute forward conversion tolls of a given fiat pair.
The forward exchange rate is simply an anticipated conversion toll for the future. In contrast, the spot ratio is simply the prevailing currency conversion tariff.
Financial institutions offer forward rates ranging from those that might be applicable for a week to as far as five years. In contrast, the spot duties are constantly changing and quoted using bid and ask spreads.
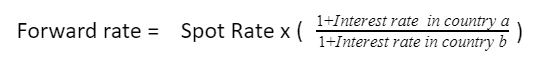
How it works
Consider a prevailing conversion tariff between the pound sterling and the greenback of (£0.75/$1). Now, consider that the UK offers 3% in interest duty while the US offers 5%.
If we converted $1,000 to the pounds, we would receive £750. Investing the amount in a British account for one year, we would end up with £772.
However, instead of swapping the amount into pounds from the 3% duty, we could simply invest the $1000 in an American account to take advantage of the 5% interest on offer and then afterward convert the amount to British pounds.
The tariff that will be used in the future would, in this case, be a forward exchange levy which is computed using the difference in borrowing levies.
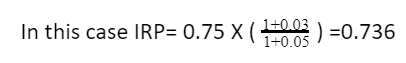
Now, if we invest the initial $1,000 in a US bank for 5% for a year, at the end of the year, we would end up with $1050. We would then convert the $1050 into pounds using the forward exchange of 0.736. In this case, we would end up with £772.80, the same amount if we had converted our money to the British pound and deposited it to take advantage of the 3% lending duty on offer.
Covered vs. uncovered IRP
IRP is said to be covered if there is no mention of contracts touching on forward interchange rates. In this case, any parity is ascertained by using the expected conversion toll. In contrast, a forward exchange rate is a major component when it comes to covered IRP.
However, there is no major difference between the two, given that there is no major difference between the spot and the forward spot tariff. The only major difference is that when dealing with covered, one is simply locking future tariffs, while with uncovered, one is simply forecasting what the tariffs will be.
Covered interest rate arbitrage
There are scenarios in the currency market where two countries offer different lending duties, but their legal tenders trade at par, for instance, legal tender X = Legal tender Y. With covered interest arbitrage, a trader or an investor could look to profit from the difference in bank rates between the two equal legal tenders.
If legal tender X comes with a 3% lending rate and Currency Y 5%, one can borrow currency X at 3% and convert the amount to Currency Y to take advantage of the higher banking charge.
In return, one can use a one-year forward rate to avert the interchange rate risk that might come into play on holding legal tender Y.
Bottom line
Having a clear understanding of how to calculate forward interchange rates is important. In addition, it is crucial to master covered and uncovered IRP.
For instance, any person leveraging covered IRP should always take into consideration the prevailing benchmark tariffs between two countries. In addition, one should always avoid the temptation of simply borrowing in a country with low lending costs to invest in one that offers lending duty, given the interchange risk that could come into play. However, one can still borrow in a country with low bank rates to invest in assets that promise higher returns.
In contrast, uncovered IRP dictates that the difference between two nation’s lending rates will always be the same as the expected change in conversion tariffs between their legal tenders. In this case, if the bank tariff difference is 2%, there is always a high probability of a currency with the highest price of money depreciating by about 2% in the long run.
